Is Subtraction Associative For Natural Numbers
Just one example to show its not true. Not a natural number.

Properties Of Integers Closure Commutative Associative Distributive
Thus subtraction doesnt have the associative property.

Is subtraction associative for natural numbers. Your question isnt gibberish but it is the wrong way round all the same. However consider the intuitive notion of subtraction where one simply performs addition with the additive inverse of b. 4 rows 6-2 -3.
10 8 3 10 5 5 10 8 3 2 3. If a b and c are the three whole numbers then a b c a b c. On the other hand the associative property does not hold good for subtraction and division of natural numbers.
Subtraction of two whole or natural numbers does not always result in a whole number or natural number. Its not impossible for subtraction to be associative though it definitely isnt true for the natural numbers. Associative property can only be used with addition and multiplication and not with subtraction or division.
If we subtract the first two numbers 10 minus 5 it gives us 5. 3 1 15 45 3 1 15. N is closed associative and commutative under both addition and multiplication but not under subtraction and division.
There is no such thing as generic subtraction only subtraction in a particular algebraic system and you have rightly identified the system you have in mind the natural numbers. 2 15 1. Download FREE Study Materials.
The associative property holds true. -6 is not a naturalwhole number. 3The set of integers under subtraction is not a group because it does not satisfy all of the group PROPERTIES.
Example of Associative Property for Addition. Contrary to addition subtraction doesnt have the associative property. The difference between any two natural numbers need not be a natural number.
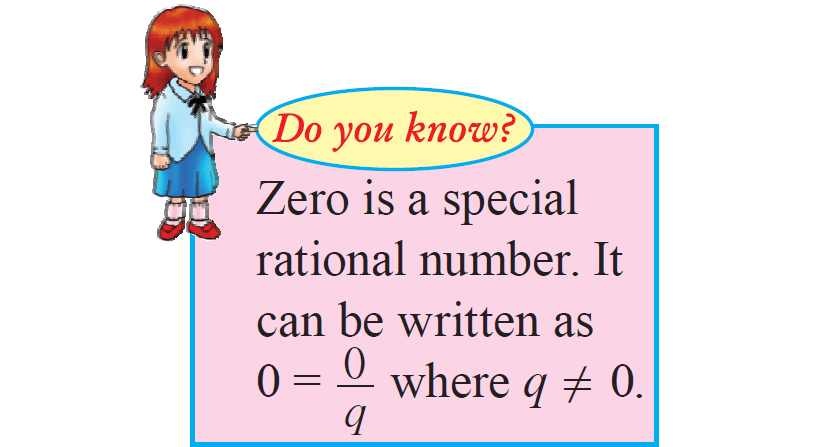
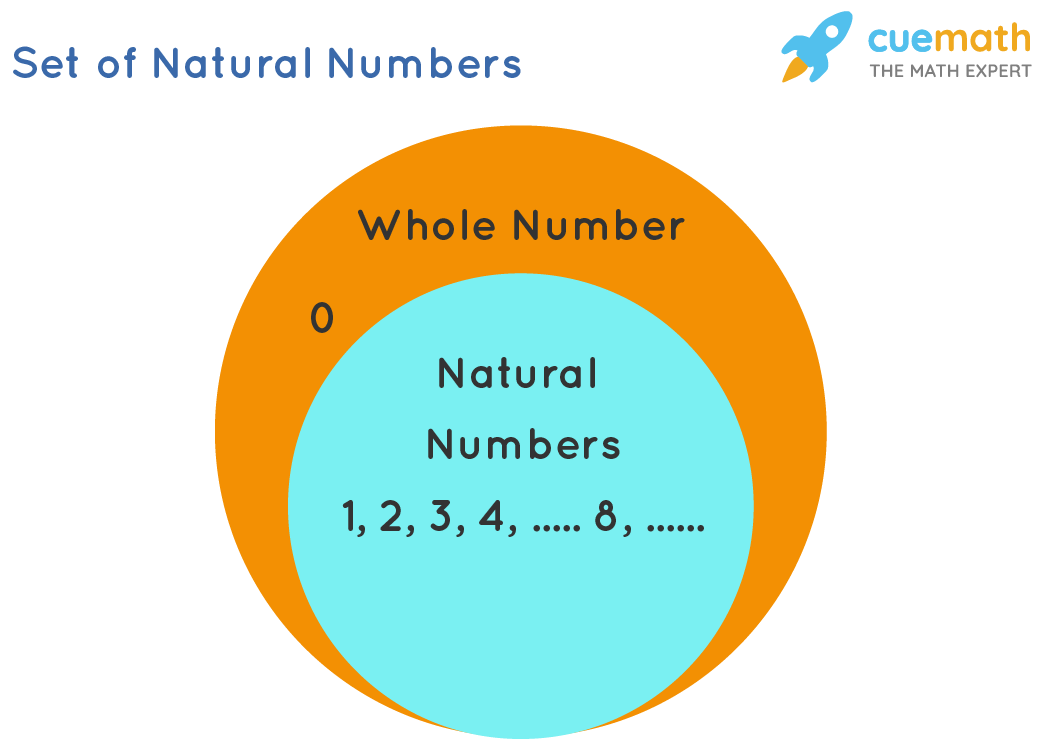
Negative numbers fractions and decimals are neither natural numbers nor whole numbers. Multiplication a b c a b c Take a 12 b 32 c 52 LHS a b c 123252 123 52 2 12154 1 152 4. 0 is not a natural number it is a whole number.
Changing the way of associating the numbers in subtraction changes the answer. Order of subtraction is an important factor. A Natural numbers are associative for subtraction b Whole numbers are not associative for subtraction c Integers are associative for subtraction d Rational numbers are associative for subtraction.
The associative property is true in the case of addition and multiplication of natural numbers. For example 1 and 2 are natural numbers. Now 3 9 -6.
Associative property involves 3 or more numbers. This means that we cannot group any two whole numbers and subtract them first. Subtraction is essentially always an inverse operation to addition not because of anything deep but.
If we assume any two natural numbers their sum and the product are also the natural numbers. Heres a more interesting example. For example take any two natural numbers say 3 and 9.
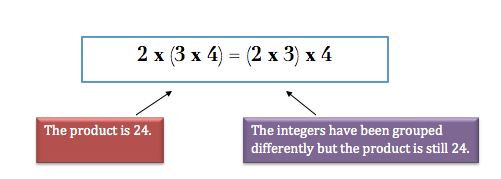
For natural numbers a b and c multiplication is associative which means a b c a b c. You cant do that in the natural numbers. Since the first case of the definition of addition is identical to the definition of addition of natural numbers from 11 the two additions give the same result when applied to two natural numbers.
That is the sum or product of any three natural numbers remains the same even if the grouping of numbers is changed. It does not have the ASSOCIATIVE PROPERTY see the previous lectures to see why. The numbers that are grouped within a parenthesis or bracket become one unit.
An associative property does not hold for the subtraction of whole numbers. Therefore the set of integers under subtraction is not a group. A b b a If one uses the intuition of add the negative of the number as a means of subtraction you are simply using addition a commutative operation.
The set of natural numbers is closed under which of the operations. Therefore the system is not closed under addition. Hence N is not closed under subtraction.
For all integers aand b. Subtraction For three natural numbers a b and c subtraction is not associative meaning a b c is not equal to a b c. Subtraction is not associative.
10 4 3 10 1 9 10 4 3 6 3 3. The associative and commutative laws of addition can now be proved for. But their product and quotient dont need to be the natural numbers.

Properties Of Natural Numbers Ppt Download

Properties Of Natural Whole Numbers Concepts Videos And Examples

Properties Of The Number Systems

What Is Natural Number Example Properties Faq S

Properties Of Natural Whole Numbers Concepts Videos And Examples
What Are Natural Numbers Definition Examples And Facts

Properties Of Rational Numbers Concepts Videos And Solved Examples

Prepare A Table For Closer Property Commutative Property Associative Property For Addition Brainly In

Commutative Law Of Addition Video Khan Academy
Property Associative Property Of Integers Under Addition
The Properties Of Real Numbers

Properties Of Whole Numbers Addition Subtraction Multiplication Division

Maths Associative Property Of Rational Numbers English Youtube
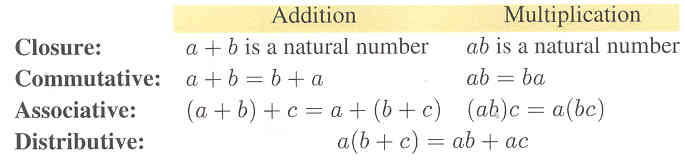
Section 5 1 Natural Numbers The Nature Of Mathematics 13th Edition

Natural Numbers Concepts Properties And Examples

Properties Of Real Numbers Ppt Video Online Download

Associative Property Does Not Work With Subtraction Youtube

07 Associativity For Rational Numbers Youtube