Multiplicative Inverse Matrix Generalized Form
The video explains what an id. The inverse matrix A 1 can be designated as.

9 4 Multiplicative Inverses Of Matrices And Matrix Equations Flashcards Quizlet
R4 A21R3 5.

Multiplicative inverse matrix generalized form. X21 R6R2 9. In math symbol speak we have A A sup -1 I. R1 A1 11 2.
A True b False. In mathematics a multiplicative inverse or reciprocal for a number x denoted by 1x or x1 is a number which when multiplied by x yields the multiplicative identity 1. Form an upper triangular matrix with integer entries all of whose diagonal entries are 1.
13 Let us notice that the matrix R5 in the relations 13 is equal to the minus. A AA-1 A-1A I. Reverse order of multiplication and must by symmetry also be equivalent to 3 4 5 and 6 Now X XXA XX A AY XAY XAAYY AYY Y.
For the multiplicative inverse of a real number divide 1 by the number. R5 R4 A22 6. Left begin array cccc2 1 1.
Multiplicative inverse matrix generalized form to solve is which of the following. Now one formula for finding the inverse of the matrix is. B AA-2 A-3A I.
The product of a matrix A and its inverse A 1 must equal the identity matrix I for multiplication. Is the identity matrix for multiplication for any second-order matrix. 9 people answered this MCQ question is the answer among for the mcq Multiplicative inverse matrix generalized form to solve is.
This MATHguide video demonstrates how to calculate the multiplicative inverse of a matrix both by hand and using a calculator. Note tha At need not be a square matrix and may even be zero. D AA-1 2A-5A Q.
The unique solution of 3 4 5 an d 6 will be called the generalized inverse of A abbreviated gi and written X A. Reduce the left matrix to row echelon form using elementary row operations for the whole matrix including the right one. By using this website you agree to our Cookie Policy.
Multiplicative inverses exist for some matrices. Find the multiplicative inverse of 8 mod 11 using the Euclidean Algorithm. The inverse of a matrix exists if and only if it is a non-singular matrix.
This tells you that. R7 R3X21 10. We observe that this matrix equation is in the standard form Axb where A beginpmatrix231016endpmatrix.
From the previous matrix equation two systems of linear equations can be written as. The multiplicative inverse of a fraction ab is ba. Suppose A is equal to a nonzero matrix of second order.
Subtract integer multiples of one row from another and swap rows to jumble up the matrix keeping the determinant to be 1. R3 R1A12 4. Matrix which does not have an inverse by solving it is classified as which of the following.
The multiplicative inverse of a matrix is the matrix that gives you the identity matrix when multiplied by the original matrix. The usual matrix inverse is defined as a two-side inverse ie AA 1 I A 1 A because we can multiply the inverse matrix from the left or from the right of matrix A and we still get the identity matrix. Well organize our work carefully.
X11 R1 R7 11. First we need to find the determinant of this matrix which is. C 3AA4 A-1A I.
Using the result A 1 adj Adet A the inverse of a matrix with integer entries has integer entries. Well do the Euclidean Algorithm in the left column. Generalized matrix inversion is not harder than matrix multiplication 3 using the temporary matrices R1R7 and the following relations 15.
11 81 3 3 11 81 8 32 2 2 8 32. Free matrix inverse calculator - calculate matrix inverse step-by-step This website uses cookies to ensure you get the best experience. R2 A21R1 3.
To find the inverse matrix augment it with the identity matrix and perform row operations trying to make the identity matrix to the left. I will use the determinant method. Multiplicative inverse matrix generalized form to solve is which of the following.
I then work through three examples finding an Invers. Then to the right will be the inverse matrix. So augment the matrix with the identity matrix.
There are a couple of ways to do this. A AA-1 A-1 A I b. It will verify that gcd811 1.
Set the matrix must be square and append the identity matrix of the same dimension to it. X12 R3R6 8. R6 R1 5 7.
For a matrix in the form. Substituting in our values we find the determinant to be. Then well solve for the remainders in the right column before backsolving.
Up to 8 cash back THE MOORE-PENROSE INVERSE Row Reduced Echelon Form and Matrix Equivalence The Hermite Echelon Form Full Rank Factorization The Moore-Penrose Inverse Solving Systems of Linear Equations Schur Complements Again GENERALIZED INVERSES The 1-Inverse 12-Inverses Constructing Other Generalized Inverses 2-Inverses The Drazin Inverse The Group Inverse. For example the reciprocal of 5 is one fifth and the reciprocal of 025 is 1 divided by 025 or 4. As a result you will get the inverse calculated on the right.
To calculate inverse matrix you need to do the following steps. I start by defining the Multiplicative Identity Matrix and a Multiplicative Inverse of a Square Matrix.

Multiplicative Inverses Of Matrices And Matrix Equations Video Lesson Transcript Study Com

9 4 Multiplicative Inverses Of Matrices And Matrix Equations Flashcards Quizlet

9 4 Multiplicative Inverses Of Matrices And Matrix Equations Flashcards Quizlet

9 4 Multiplicative Inverses Of Matrices And Matrix Equations Flashcards Quizlet

Chiang Wainwright Mathematical Economics Chapter 4 Linear Models

Multiplicative Inverses Of Matrices And Matrix Equations Video Lesson Transcript Study Com
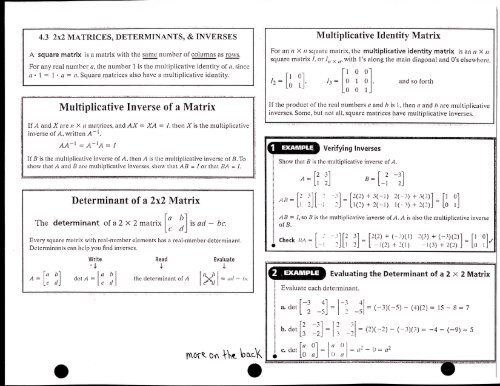
Multiplicative Inverse Of A Matrix Determinant Of A 2x2 Matrix

9 4 Multiplicative Inverses Of Matrices And Matrix Equations Flashcards Quizlet

9 4 Multiplicative Inverses Of Matrices And Matrix Equations Flashcards Quizlet

Pin On Math Classroom Activities
Multiplicative Inverses Of Matrices And Matrix Equations Video Lesson Transcript Study Com

Matrix Inverse From Wolfram Mathworld

Linear Algebra Learning The First Chapter And The Matrix Equations 1 3 Matrix Arithmetic Programmer Sought

9 4 Multiplicative Inverses Of Matrices And Matrix Equations Flashcards Quizlet




