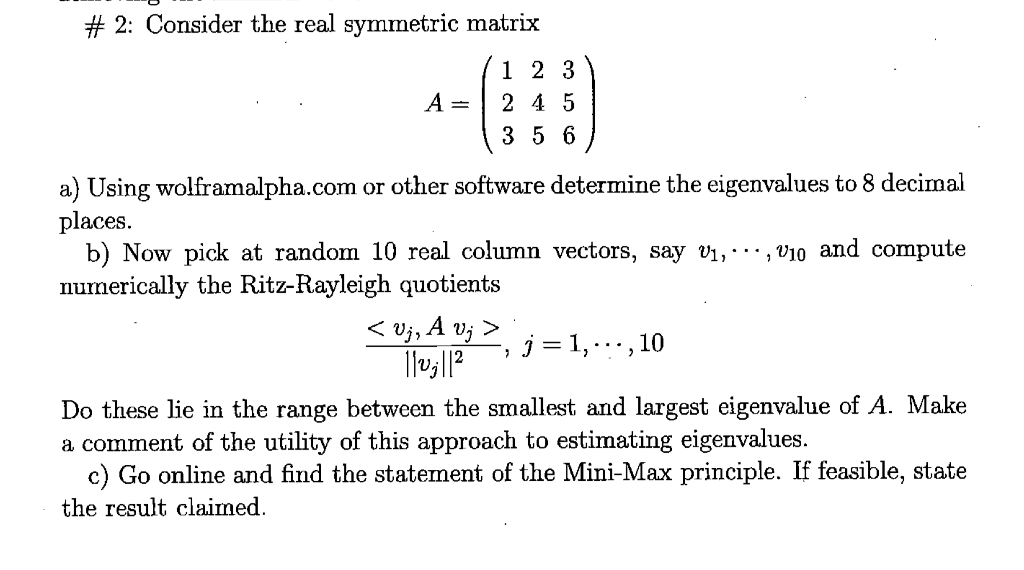
Symmetric Matrices Have Eigenvalues
U Tu u TAu u TAu ATu Tu since BvT vTBT. Symmetric matrices A symmetric matrix is one for which A AT.
Karl Stratos Research Eigentutorial
Let Aai li j.
Symmetric matrices have eigenvalues. A nxn symmetric matrix A not only has. Thus distinct eigenspaces of a skew symmetric matrix are orthogonal. The general proof of this result in Key Point 6 is beyond our scope but a simple proof for symmetric 22matrices is straightforward.
The matrix is symmetric and its pivots and therefore eigenvalues are positive so A is a positive definite matrix. A λI 2 λ 8λ 11 0 ie. Furthermore the a with i i are required to have the same distribution function 4 while all the ú7í possess the same distribution G.
Its a Markov matrix its eigenvalues and eigenvectors are likely. Negative definite Hermitian symmetric matrix must have all strictly neg-ative eigenvalues. If Aisareal symmetricmatrix thenall of its eigenvalues arereal andit hasareal eigenvectorie.
Prove that all the eigenvalues of A are real numbers by considering the characteristic polynomial of A. 2 I Now we pre-multiply 1 with u T to obtain. So if a matrix is symmetric--and Ill use capital S for a symmetric matrix--the first point is the eigenvalues are real which is not automatic.
More generally a Hermitian matrix that is a complex matrix equal to its conjugate transpose is positive-definite if the real number is positive for every nonzero complex column vector where denotes the conjugate. A symmetric matrix A is a square matrix with the property that A_ijA_ji for all i and j. Eigenvalues of 2 2 Symmetric Matrices are Real by Considering Characteristic Polynomials Problem 609 Let A be a 2 2 real symmetric matrix.
For Ax λx. Positive definite matrices are even bet ter. A u u ie Au u.
The eigenvalues of A are real numbers. Do your results agree with Theorem 571. And since it must be that and so and are orthogonal.
1 I Taking complex conjugates of both sides of 1 we obtain. 52 Quadratic Forms motivating quote from David Lays Third Ed Linear Algebra and Its Applica-tions. The eigenvalues of a symmetric matrix with real elements are always real.
But its always true if the matrix is symmetric. Hence the eigenvalues of a skew symmetric matrix are real. Its eigenvalues are the solutions to.
In mathematics a symmetric matrix with real entries is positive-definite if the real number is positive for every nonzero real column vector where is the transpose of. There is a very important class of matrices called symmetric matrices that have quite nice properties concerning eigenvalues and eigenvectors. Eigenvalues of a symmetric real matrix are real I Let 2C be an eigenvalue of a symmetric A 2Rn n and let u 2Cn be a corresponding eigenvector.
A symmetric matrix has real eigenvalues. Symmetric Hermitianindefintematrix is one that has some positive andsome negative and possibly zero eigenvalues. If we transpose this we find that xTAλxT note that A AT.
1 Eigenvalues of a real symmetric matrix are real. The determinant of a positive definite matrix is always positive but the de. Show that the following symmetric matrices are positive definite and calculate their eigenvalues.
Let rr4A be the eigenvalues. Symmetric matrices are good their eigenvalues are real and each has a com plete set of orthonormal eigenvectors. So it too is invertible.
The rst step of the proof is to show that all the roots of the characteristic polynomial of Aie. Multiply the first equation on the left by. But A is real so it equals its own conjugate and hence Axλx.
For real vectors it is the usual dot product v w v w. In symmetric matrices the upper right half and the lower left half of the matrix are mirror images of each other about the diagonal. The matrices are symmetric matrices.
Returning to the case with now known to be real and so Equation becomes. And the second even more special point is that the eigenvectors are perpendicular to each other. Those are beautiful properties.
If a matrix has some special property eg. Different eigenvectors for different eigenvalues come out perpendicular. Symmetric matrices have real eigenvalues The Spectral Theorem states that if Ais an n nsymmetric matrix with real entries then it has northogonal eigenvectors.
Extend the dot product to complex vectors by v w i v i w i where v i is the complex conjugate of v i. If we use complex conjugates Axλx.
Understanding A Proof Eigenvalues Of A Real Symmetric Matrix Are Real Mathematics Stack Exchange

Similar Diagonalization Of Real Symmetric Matrix Ppt Video Online Download
5 1 Eigenvalues And Eigenvectors Ppt Video Online Download
The Eigenvalues And Corresponding Eigenvectors For A Chegg Com

Linear Algebra 101 Part 7 Eigendecomposition When Symmetric By Sho Nakagome Sho Jp Medium
Http People Stat Sfu Ca Lockhart Richard 350 08 2 Lectures Theory Web Pdf

Symmetric Matrices Eigenvalues Eigenvectors Youtube
Eigenvalues And Eigenvectors Of Symmetric Matrices Linear Algebra

Chapter 7 Symmetric Matrices And Quadratic Forms Flashcards Quizlet

Orthogonal Matrix Of Symmetric Matrix Eigenvectors Mathematics Stack Exchange
Eigenvalues And Eigenvectors Of Symmetric Matrices Linear Algebra
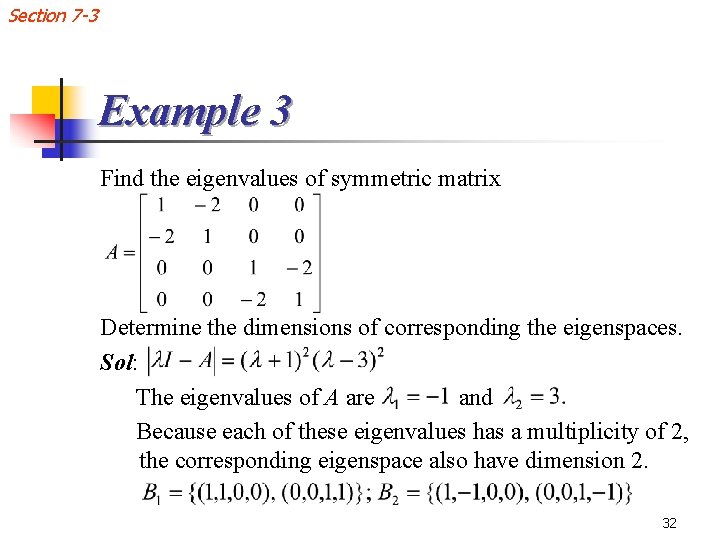
Eigenvalues Eigenvectors 7 1 Eigenvalues Eigenvectors N N

Symmetric Matrices And Quadratic Forms Ppt Download

What Are The General Form Of Eigenvalues Of An Orthogonal Matrix Mathematics Stack Exchange
Http Www Cse Iitm Ac In Vplab Courses Larp 2018 Chap5 Evv Larp Pdf
Karl Stratos Research Eigentutorial

Real Symmetric Matrices Have Only Real Eigenvalues Is This An Incorrect Proof Mathematics Stack Exchange

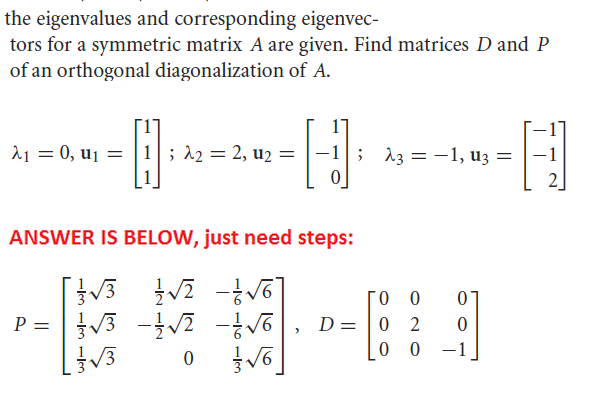
2 Consider The Real Symmetric Matrix 1 2 3 A Chegg Com
Karl Stratos Research Eigentutorial

