Consider The Matrices A B C And D With Order
2d 11 3. Consider the matrix ABCD A B C D with order 2 3344442 2 3 3 4 4 4 4 2 respectively.
Order Of A Matrix Determine The Order Of Matrix Solved Examples
Let x kABC2D3 x k A B C 2 D 3.
Consider the matrices a b c and d with order. -1 0 1 10 pts Find if possible the values of x y and z such that. We answer the question whether for any square matrices A and B we have A-BABA2-B2 like numbers. D 82 d 4.
A b c d 1 adbc1 d b c a. Consider two matrices 2D arrays A and B of integer numbers each with n rows and n columns. Now quadrilateral ABCD in the matrix form can be represented as 2 4 A B C D 1 3 1 1 X 0 2 3 2 or 4 2 A 1 0 B 3 2 Y C 1 3 D 1 2.
Let x alpha A B gamma C2 D3 where alpha and gamma are scalars. D This matrix is Hermitian because all real symmetric matrices are Hermitian. SL2R n A GL2R detA 1 o Suppose AB SL2R.
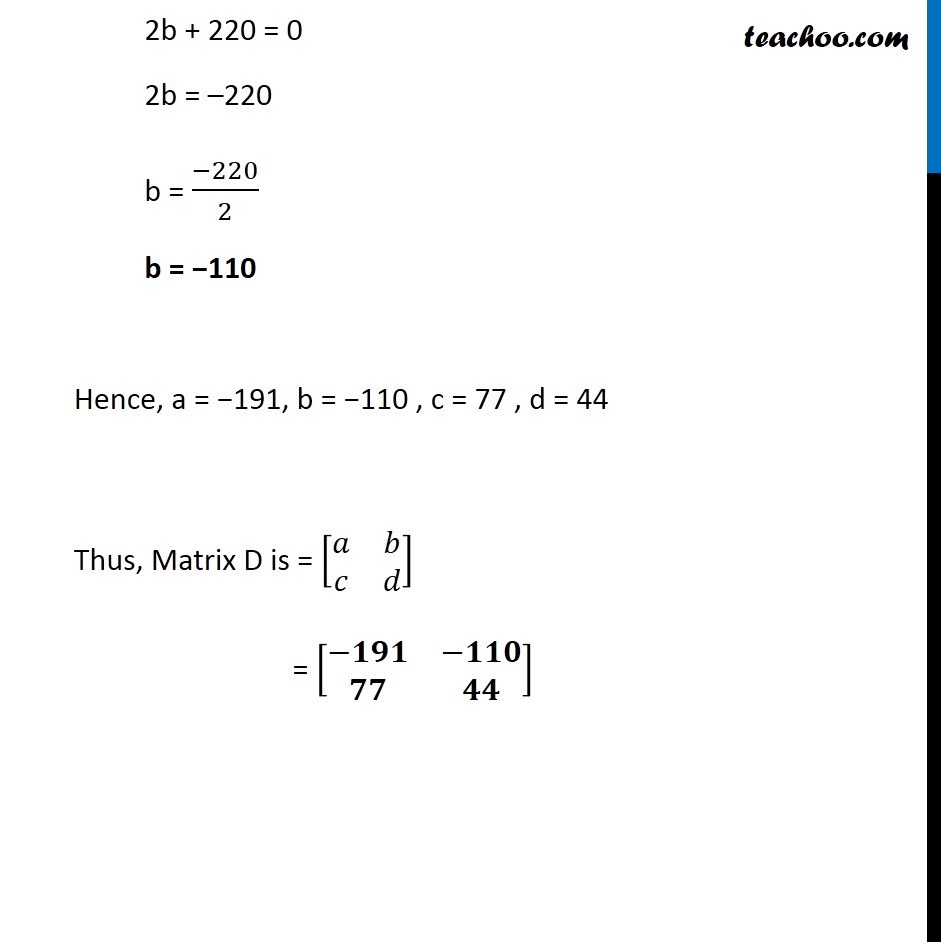
Consider the matrix A B C D with order 2 3 3 4 4 4 4 2 respectively. 2 2c 14. On the other hand the matrix 2 1 1 2 is not an element of GL2Z3.
A BT C. 3 2d 11. For example consider a quadrilateral ABCD with vertices A 1 0 B 3 2 C 1 3 D 1 2.
Thus 2 1 2 2 1 21 2 2 1 2 2 cdot 2 2 1 2 1 1 2 1. Show that if A is 2x2 then the first theorem gives the same formula for A1 as that given by the second theorem. Click hereto get an answer to your question Consider the matrix A B C D with order 2 3 3 4 4 4 4 2 respectively.
D and o Work Problem 2 20 points. 2a 18 4 14. 1 a b ad be cd afd 0 1 d e fd 0 0 1 f from which we see directly that the matrix is invertible if all a d and f are different from zero.
If a 0 p 1 cases and d 0 one case and b 0 p 1 cases then c must be 0 so this yields p 1 p 1 p 2 2 p 1 tuples. For the product AB the inner dimensions are 4 and the product is defined but for the product BA the inner dimensions are 2 and 3 so the product is undefined. 4 2a 18.
Then the value of k. B C 0. C 122 c 6.
If A a b c d and adbc0 then A is invertible and the inverse is 1adbc d -b -c a. Then the value of k. C This matrix is Hermitian.
It is not too difficult to solve directly a b c 0 d e 0 0 fx y z 0 y v 0 0 w 1 0 0 0 1 0 0 0 1 giving. A 142 a 7. 0 1 1 0 3 0 0 3 1 1 0 0 2 0 2 2 D C B A Which of the above matrices are each of the following.
1 2b 7. The determinant is a polynomial in. B This matrix is symmetric but not Hermitian because the entry in the first row and second column is not the complex conjugate of the entry in the second row and first column.
A b c d Solution a This matrix is not Hermitian because it has an imaginary entry on its main diagonal. Consider the following matrices. Consider the following in respect of matrices A B and C of same order.
Let V2 V2 A 2 2 be. 2b 7 1 6. Let x k ABC2 D3.
B 62 b 3. Let x α A B γ C 2 D 3 where α and γ are scalars. We want to calculate C AB where C is another nn matrix.
Your answer maybe more than one a. 1 Write a code in CJava for the function that calculates and displays C. 2 n 2 1 A 2 2 n 2 1 A 1 1 2 n 2 A 2 n 2 So B C B C O dt.
Scalar 3 marks 2. Then the value of k k. Let x k A B C 2 D 3.
Consider the 2 y matrices A 2 y 24 -2 1 C where x y and z are real numbers. Yes consider a matrix A with dimension latex3times 4latex and matrix B with dimension latex4times 2latex. If Awas a 3 by 3 matrix we would see a polynomial of degree 3 in.
2 n 1 A 2 2 n 1 A 1 2 n 1 A 2 n 1 1 A 2. DetA I 2 a d ad bc 0 trA detA This polynomial is called the characteristic polynomial. It has determinant 2211 0 so its not invertible.
Figure in the form of a matrix. C d A I a b c d. AC I2 where I2 0 Work Problem 3 20 points.
This polynomial is important because it encodes a lot of important information. Determine the matrices A and B when. If a 0 p 1 cases and d 0 one case and b 0 one case then c is arbitrary p cases so this yields p 1 p p 2 p tuples.
Ii 10 pts Find if possible the values of x and y such that. Therefore a 7 b 3 c 6 and d 4. 1 A B C A B C 2 AB AB 3 ABC CBA where A is the trans.
2 Then analyze the time complexity of your algorithm in the worst-case big-O with clear and complete explanation. Let x α A B γ C2 D3 x α A B γ C 2 D 3 where α α and γ γ are scalars. The determinant is a polynomial in of degree 2.
B A 2 n A 2. Algebra questions and answers. 2c 14 2 12.
If A is an invertible nxn matrix then the inverse of matrix A is A-1 1det A adjA. Show that the following set is a subgroup of GL2R. We actually give a counter example for the statement.
Comparing the corresponding elements we have. Hence a 2b 4 c 1 and d 3.
25 Motivational Eric Thomas Quotes Next Level Gents Eric Thomas Quotes 25th Quotes Eric Thomas
Pin On 10 Math Problems
Pin On School Literature
Pin On Math Videos
Diagonalization
Pin By Rehana Ghafar On Mathematics Column Mathematics Matrix
Example 28 Find A Matrix D Such That Cd Ab O Examples
Cramer S Rule With Three Variables Chilimath
What Is An Identity Matrix Studypug
Https Web Northeastern Edu Suciu U371 U371sp06 Sol7 Pdf
Pin On Math
Education By Lynnette On Indulgy Com Literacy Strategies Struggling Readers Reading Strategies
Pin On Ncert Solutions Class 12 For Maths
4 Multiplication Of Matrices
Example 28 Find A Matrix D Such That Cd Ab O Examples
Pin On Management
Determinant Of A Matrix Linear Algebra Using Python Algebra Python Linear
Pin On 10 Math Problems
Example 28 Find A Matrix D Such That Cd Ab O Examples
