Solving Matrices With Complex Numbers
Sort complex numbers into complex conjugate pairs. We know how to multiply two polynomials and so we also know how to multiply two complex numbers.
Pin On Imaginary Numbers Properties And Operations
In this video well learn how to view a complex number as a 2x2 matrix with a special form.
Solving matrices with complex numbers. Here you can solve systems of simultaneous linear equations using Gauss-Jordan Elimination Calculator with complex numbers online for free with a very detailed solution. The determinant of the matrix representation of a complex number corresponds to the square of its modulus. Email me if my answer is selected or commented on.
So a multiplication of an element of and a number gives a matrix in. 3 14 i 4 5 i. This is accomplished by simply conjugating one of the vectors.
The trick here is to multiply be the conjugate giving. The Real Statistics Resource Pack supplies the following array functions where Y and Z are ranges that represent complex matrices while z is a range that represents a complex scalar number. The transpose of the matrix representation of a complex number corresponds to complex conjugation.
In other words if we add two elements of the set we still get a matrix in. In other words it is the original complex number with the sign on the imaginary part changed. Learn more about complex numbers linear equations linear systems linear complex symbolic unnecessarily symbolic MATLAB Symbolic Math.
The linear equation solver can solve any m n linear equations. How to solve 2 systems of linear equations with. Matrix AdditionSubtraction Complex numbers A complex number is a number that can be expressed in the form a bi where a and b are real numbers and i.
Addition subtraction and multiplication of complex numbers can be naturally defined by using the rule i2 1 combined with the associative commutative and distributive laws. Adding and multiplying complex numbers corresponds to adding or multiplying their matrix representations. This makes the complex numbers a field that has the real numbers as a.
Thus the second element of the second row now reads. In particular we have. Z z T z.
All we need to do is foil the two complex numbers to get 4 5 i 12 11 i 48 44 i 60 i 55 i 2 48 16 i 55 i 2 4 5. Every nonzero complex number has a multiplicative inverse. Determine whether array uses complex storage.
Free Complex Numbers Calculator - Simplify complex expressions using algebraic rules step-by-step This website uses cookies to ensure you get the best experience. This complex matrix calculator can also perform matrix algebra all the previously mentioned matrix operation s and solving linear system s with complex matrices. Imaginary part of complex number.
MultiplicationFor any real numbers. Well also see that there is a matrix version for the number 1 a. The fix as you have probably guessed is to sum the squares of the magnitudes of the components of z.
Here are some examples of complex numbers and their. I want to know how to solve a matrix with complex numbers in a TI-84 plus calculator. Answered Mar 16 15 at 1142.
Essentially I am starting with the RHS of this equation. Essentially we are expressing a complex matrix as A Bi where A and B are matrices that only have real values. Namely we define the length of a complex vector via.
Real part of complex number. Matrices can have real imaginary and in general complex numbers as their elements. Matrix addition subtraction multiplication and inverse on complex matrices are calculated using only real matrix operations as follows.
In the example above this produces. Multiplication by a numberWe have. Asked Sep 6 2013 by anonymous.
Email me at this address if my answer is selected or commented on. 1 day agoI have a 1-row matrix of complex numbers in polar form which represent the difference of 3 other numbers I am solving for. Absolute value and complex magnitude.
So thinking of numbers in this light we can see that the real numbers are simply a subset of the complex numbers. 3 14 i 4 5 i 3 14 i 4 5 i 4 5 i 4 5 i 82 41 i 41 2 i. The conjugate of the complex number a bi is the complex number a - bi.
3 2 i 2 2 i 2 i 3. Our calculator is capable of solving systems with a single unique solution as well as undetermined systems which. Sign function signum function unwrap.
Pin On Mathematics
Pin On Matrices
Pin On Alg 2 Trig
Solving Equations With Math Assistant In Onenote In 2021 Solving Equations Math Graphing
Pin On Education Teaching Ideas
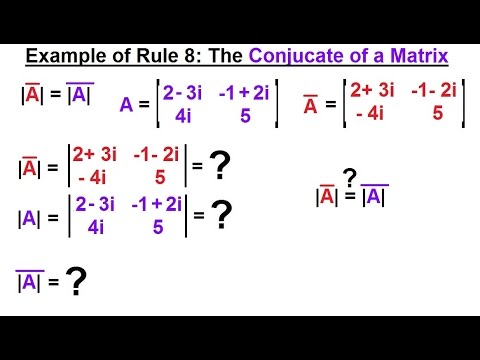
Linear Algebra Ch 2 Determinants 14 Of 48 Example Of Rule 8 The Conjugate Of A Matrix Youtube
Pin On Math Aids Com
Pin On Mrs Algebra
Pin On Grade 12 Eureka Math
Pin On Education Worksheets Template
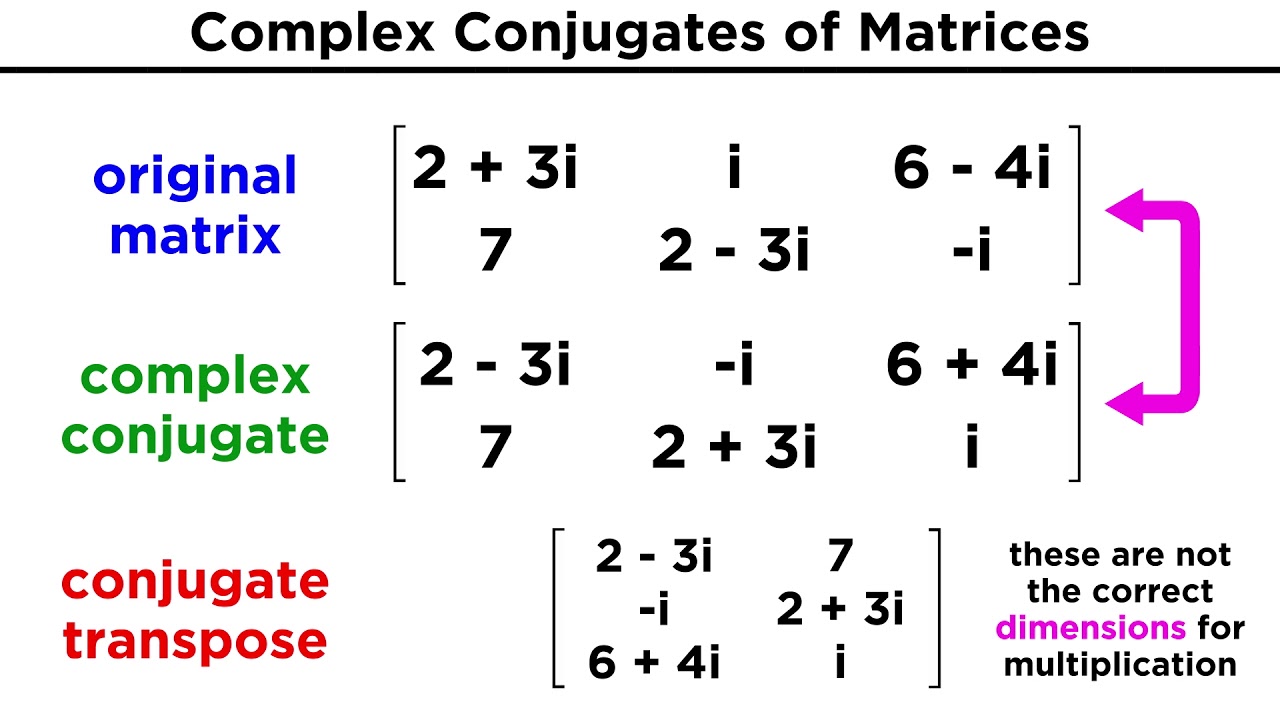
Complex Hermitian And Unitary Matrices Youtube
Pin On Education
Pin On Math
Pin On Algebra 2
Pin On Programming
Pin On Math Aids Com
Well Multiplying A Matrix With Number Such As Two Is Very Easy This Kind Of Matrix Multiplication Is Called Matrix Multiplication Multiplication Real Numbers
Pin On Teaching Math
Pin On Precalculus Curriculum